
Insights from using biplanar intersection for freehand frontal ventriculostomy: a retrospective case-control study with virtual simulation
Introduction
Frontal ventriculostomy (FV), also known as frontal-horn lateral ventricular puncture (LVP), is a widely used method for managing lateral ventricular hemorrhage or obstructive hydrocephalus (1). The success rate of this procedure is crucial in predicting the patient’s outcome and the likelihood of related complications (2,3). Kocher’s puncture is a well-known and widely accepted technique for performing FV (4-8). However, the failure rate of Kocher’s puncture performed manually varies significantly, with rates ranging from 6.5–28.5% across various studies (4,7-12). Attempting subsequent punctures after an initial failed needle insertion significantly increases the risk of post-puncture complications such as hemorrhage and edema (3,9,13).
The advent of stereotactic or neuronavigation technology has significantly enhanced the precision of FV (14-18); however, in emergency situations, the freehand technique has distinct advantages, as it can be executed rapidly without costly equipment (10,19-21). Traditional FV methods primarily rely on surface anatomical landmarks for guidance, including the external acoustic meatus (EAM), ipsilateral medial canthus (IMC), nasion, and contralateral medial canthus (CMC). These landmarks are susceptible to individual, regional, and racial variations, which might contribute to the less than optimal success rates of FV procedures (22-24).
Thus, a rapid and precise method for LVP needs to be established. Fortunately, with the continuous improvement of three-dimensional (3D) imaging reconstruction technology and software, there are new opportunities to enhance the success rate of freehand FV. One such opportunity lies in the use of virtual models to analyze FV methods. Notably, the biplanar intersection (BI) method, which uses the mathematical principle of two intersecting planes, represents a promising approach. By applying this BI technique to stereotactic brain puncture, and reverse-engineering the FV process using 3D reconstruction, we can gain a deeper understanding of the mechanics involved in frontal-horn puncture. To date, no relevant literature on this subject appears to have been published. We present this article in accordance with the STROBE reporting checklist (available at https://qims.amegroups.com/article/view/10.21037/qims-24-1381/rc).
Methods
Study design and population
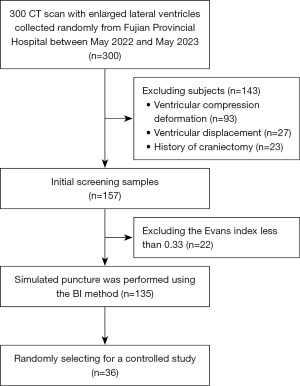
This retrospective case-control study randomly selected 300 data from cranial computed tomography (CT) scans acquired at Fujian Provincial Hospital between May 2022 and May 2023. Patients were included in this study if they were aged ≥18 years, presented with lateral ventricular enlargement (a width >10 mm), and had an Evans index >0.33. The Evans index, a pivotal diagnostic criterion for hydrocephalus, is the ratio of the frontal-horn width to the skull diameter. Patients with ventricular compression, deformation, displacement, or those who had undergone bone flap decompression surgery were excluded from the study. In total, 135 patients were sure (Figure 1). To facilitate accurate data analysis, only CT scans with a uniform thickness of <2 mm were included in the analysis. The study was conducted in accordance with the Declaration of Helsinki (as revised in 2013). The study was approved by the Ethics Committee of the Ethics Committee of Fuzhou University Affiliated Provincial Hospital (No. K2020-06-019), and the requirement of individual consent for this retrospective analysis was waived.
Procedure
3D reconstruction of craniocerebral model
CT images in Digital Imaging and Communication in Medical format were imported into the 3D-Slicer software (version 4.11, Belgium). Due to inconsistencies in the baseline alignment across various scans, the imaging baselines were first standardized by precisely aligning them with the orbitomeatal baseline. The midline was also adjusted to ensure bilateral symmetry. Following this standardization, the differentiation of anatomical structures was achieved by leveraging variations in grey-scale values. Specifically, the dynamic adaptive region growing method was employed to accurately isolate the regions of interest. These segmented regions were then transformed into virtual 3D models that effectively visualized the patients’ scalps and lateral ventricles.
Analysis of the puncture path with BI theory
The interventricular foramen (IF) was identified as the target point F-1 on the horizontal CT image. Subsequently, F-1 was laterally projected to establish projection points F-2 and F-3 on the scalp, and projected anteriorly to establish point F-4 (Figure 2A). The puncture point P was strategically positioned 20 mm anterior to the coronal suture and 30 mm lateral to the midline (Figure 2B). By connecting points P, F-2, and F-3, and points P, F-1, and F-4, respectively, two distinct planes were defined; that is, Plane 1 and Plane 2. The trajectory of the puncture was outlined by the intersection of these two planes, aligning with the line connecting points P and F-1 (Figure 2C,2D).
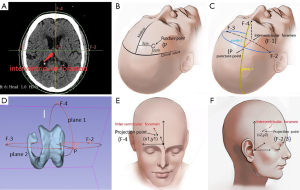
Applying the cartesian coordinate system to document projection points
The Cartesian coordinate system was employed to record the projection points. Initially, the glabella was selected as the origin of the coordinate system, with the eyebrow arch designated as the x-axis, and the midline as the y-axis. By using the curve generator tool, the coordinates of the anterior projection point were computed and documented as (x1, y1) (Figure 2E). For the lateral projection point, the intersection of the anterior edge of the tragus and the upper edge of the zygomatic arch was established as a new origin. The upper edge of the zygomatic arch was then set as the x-axis. The coordinates of the lateral projection point, denoted as (x2, y2), were measured accordingly (Figure 2F). This documentation method facilitated subsequent analysis and research.
Verifying the perpendicularity of the two planes
Figure 2D illustrates Plane 1 and Plane 2, which are defined by the points P, F-2, and F-3, and points P, F-1, and F-4, respectively. The spatial coordinates of each point were obtained using the 3D-Slicer software (version 4.11, Belgium), denoted as P1 (xa, ya, za), P2 (xb, yb, zb), and P3 (xc, yc, zc). The plane equation is given by:
The coefficients A, B, and C are determined using the following calculations:
The resulting vector (A, B, C) represents the normal vector of the plane. By applying this method to both planes, their respective normal vectors, labeled as “a” and “b,” are obtained. The angle between the two planes, denoted as θ, can be calculated using Eq. [3], which is expressed as follows:
If the measured angle approximates 90°, it can be inferred that the two planes are perpendicular to each other.
Summarizing the BI method for simulated puncture
The BI method was devised by incorporating critical puncture parameters, including the average values (x1, y1) and (x2, y2), as well as the puncture depth. This method defines the needle plane by linking Kocher’s point to the bilateral projections of the IF, determining the needle tip’s orientation by connecting Kocher’s point to the anterior projection point of the IF. To authenticate the efficacy of this approach, a computer-based reverse simulation was executed on a random selection of cases. We generated scalp-skull models and pinpointed projection locations (see Figure 2B,2C,2E,2F). Subsequently, a simulation of the frontal puncture of the lateral ventricle was conducted, which was aligned with the direction of the intersection line.
Simulated puncture with traditional method
We performed a simulated puncture procedure on a 3D virtual model employing the traditional method, which involves puncturing at Kocher’s point, and directing the needle toward the midpoint of an imaginary line connecting the bilateral EAM, ultimately pointing toward the nasion.
Evaluation metrics
Kakarla grading
This metric was used to assess the effectiveness of the FV placement in facilitating drainage. Under this grading system, the placements are categorized as follows: Grade 1 (optimal placement): placement of the tip in the ipsilateral frontal horn; Grade 2 (non-optimal placement): placement in the contralateral horn of the lateral ventricle, corpus callosum, or hemispheric fissure, resulting in retained drainage function; and Grade 3 (failed placement): placement in crucial brain regions like the brainstem or cerebellum, with or without drainage function (11).
Lateral deviation
This metric was employed to evaluate the accuracy of FV, which was defined as the distance between the achieved puncture trajectory and the IF, measured horizontally at the level of the IF.
Statistical analysis
SPSS (version 15, IBM, Armonk City, USA) was employed for the initial processing and analysis of the data. For the data that adhered to a normal distribution, the mean and standard deviation were computed. The categorical data are expressed as the frequency. For the count data, the Chi-square test was applied, depending on the specific data characteristics and research objectives. For the measurement data, the two-sided t-test was employed to compare mean differences between groups. A P value <0.05 was considered statistically significant, indicating the presence of a meaningful difference in the data.
Results
The CT data of 135 patients were collected. Of the patients, 110 were male and 25 were female. The patients had Evans grades ranging from 1 to 3. For detailed information about the patients’ demographics and Evans gradings, see Table 1. In all cases, the angles between the two planes constructed by the BI method averaged 89.6°, approximately equal to 90°, indicating that the two planes were basically perpendicular to each other, which facilitates practical manual operation.
Table 1
| Characteristics | Cases (n=135) |
|---|---|
| Sex | |
| Male | 110 (81%) |
| Female | 25 (19%) |
| Age (years) | |
| [18, 30) | 4 (3%) |
| [30, 45) | 26 (19%) |
| [45, 60) | 56 (42%) |
| ≥60 | 49 (36%) |
| Evans | |
| Grade 1 | 7 (5%) |
| Grade 2 | 8 (6%) |
| Grade 3 | 120 (89%) |
The average value of the projected point coordinates (Figure 3)
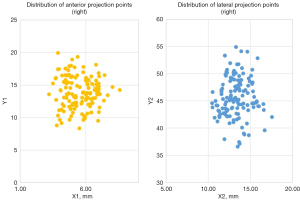
All the coordinates were defined in the Cartesian system. For the lateral projection points, the right point lied in the first quadrant at (13.3±1.5, 45.7±4.1 mm), while the left point was symmetrically located in the second quadrant at (−13.3±1.5, 45.7±4.1 mm); that is, the lateral projection point was located 13.3±1.5 mm anterior to the anterior margin of the tragus and 45.7±4.1 mm superior to the superior margin of the zygomatic arch. In relation to the anterior projection point, it was suited at (5.5±1.1, 13.8±2.4 mm), approximately 5.5±1.1 mm lateral and 13.8±2.4 mm superior to the glabella. No significant differences were observed in these measurements in terms of gender (P>0.05).
Puncture depth
The puncture depth was measured from the inner skull plate to the IF. In our study, the puncture depths ranged from 43.7 to 70.1 mm, with an average of 56.0±4.1 mm (95% confidence interval: 48.0–64.0 mm). No significant difference in the average puncture depth was observed between the male (58.0±3.8 mm) and female (56.0±2.4 mm) patients (P>0.05).
Comparison of simulated FV using the BI and traditional methods
A total of 36 cases were randomly selected for simulated FV, using both the BI and traditional puncture methods. The findings indicated that both techniques achieved successful results in all instances. However, when graded according to the Kakarla criteria, the BI method was superior (χ2=5.373, P=0.021), and had a Grade 1 rating of 100% (36/36), while the traditional method had a Grade 1 rating of 86.1% (31/36) and a Grade 2 rating of 13.9% (5/36). Further, the BI method had a significantly reduced average lateral deviation of 2.69±0.30 mm, while that of the traditional method was 13.97±0.50 mm (t=−20.61, P<0.05). Additionally, an analysis of the planes constructed using the BI method revealed that plane 1 passed between the nasal root and the CMC in 28 cases, and directly through the CMC in 8 cases, without intersecting the IMC or nasal root in any case. Meanwhile, plane 2 did not directly intersect the EAM, but passed adjacent to it (see Figure 4 and Table 2 for details).
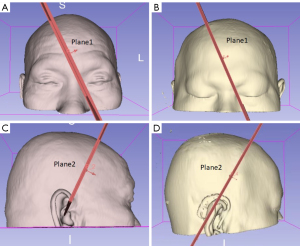
Table 2
| Evaluation metrics | BI method (n=36) | Traditional method (n=36) | P |
|---|---|---|---|
| Kakarla grading, n (%) | |||
| Grade 1 | 36 (100.0) | 31 (86.1) | 0.021 |
| Grade 2 | 0 | 5 (13.9) | |
| Grade 3 | 0 | 0 | |
| Lateral deviation (mm), mean ± SD | 2.69±0.30 | 13.97±0.50 | <0.001 |
| Plane 1 and anatomical landmarks, n (%) | |||
| Between nasion and CMC | 28 (77.8) | – | |
| CMC | 8 (22.2) | – | |
| Plane 2 and anatomical landmarks | |||
| EAM | 0 | – |
BI, biplanar intersection; SD, standard deviation; CMC, contralateral medial canthus; EAM, external acoustic meatus.
Discussion
In this study, based on the principle of BI method, with the aid of 3D models, we proposed a novel approach for the frontal-horn puncture of the lateral ventricles, termed the BI-based puncture method. Subsequently, we conducted simulated punctures for validation. Our findings showed that compared to the traditional method, the BI puncture approach provided a more precise puncture result and a higher optimal puncture rate.
The practice of employing the IF as the puncture target or reference point during FV procedure is widely acknowledged and used. Therefore, in this study, we focused on the IF as the puncture target for the BI-based puncture method. This approach derives the puncture trajectory by using the intersecting line created by two distinct planes. One plane joins Kocher’s puncture point with the lateral projection of the IF, while the other connects Kocher’s puncture point to the frontal projection. It is then able to accurately define the precise plane and direction for needle insertion. Additionally, our analysis determined an optimal puncture depth ranging from 4.8–6.4 cm (mean depth: 5.6 cm), which is close to the traditional puncture depth of 4–6 cm (25).
The primary obstacle in the BI method lies in the accurate identification of the positions of the projection points. To address this issue, we devised a Cartesian coordinate system using anatomical landmarks on the body surface to record and define the coordinates of these projection points. The results indicated that the lateral projection point is located approximately 1.5 cm anterior to the front/edge of the tragus, and 4.5 cm superior to the superior margin of the zygomatic arch. Meanwhile, the anterior projection point is situated roughly 1.5 cm above the glabella, and approximately 0.6 cm laterally. By defining these points in such a manner, their locations on the scalp can be easily pinpointed during the simulated BI puncture.
In traditional puncture methods, the selection of trajectories relies on anatomical landmarks, including the bilateral EAM, nasion, IMC, and CMC. Some studies have also proposed a puncture trajectory perpendicular to the skull that does not depend on anatomical landmarks. For example, Raabe et al. (24) simulated FV procedures, and found that specific combinations of entry points and trajectories significantly enhanced the puncture success rates; a puncture point 3–4 cm away from the midline, aimed toward the CMC, yielded a 86% Kakarla Grade 1 success rate. Conversely, perpendicular skull trajectories achieved a success rate of 83%. Points 1–2cm from the midline are best combined with a trajectory toward the nasion. This study also advised against targeting the ipsilateral inner canthus due to its low success rate. Yi et al. (26) identified an optimal entry point 9–11 cm posterior to the nasion and 2.5–3 cm lateral from the midline, and achieved a 97.79% success rate with a perpendicular trajectory. Kirkman et al. (23) noted that, despite the superiority of perpendicular and CMC/EAM trajectories over IMC/EAM in freehand anterior frontal ventricle punctures, they still have a failure rate of almost one-third. These findings underscore the inconsistencies and limitations of traditional puncture methods, highlighting the need for further research and refinement.
In our study, during the simulation of the BI-based puncture method, it became apparent that the constructed trajectories fail to strictly conform to the EAM, or uniformly align with other anatomical landmarks. Additionally, the deviation relationship between the two planes and anatomical markers was inconsistent. Notably, we found that 77.8% of the trajectories were aimed toward a point between the nasion and the CMC, echoing Park et al.’s (27) observation that optimal paths target the medial one-third distance between the midline and CMC. However, a substantial 22.2% of the trajectories in our study were directed toward the CMC, highlighting the substantial uncertainty inherent in traditional methods that rely on anatomical landmarks to determine the puncture trajectory. Thus, the high failure rate of traditional freehand puncture and the notable variations among neurosurgical centers could stem from the varying reference values of the anatomical markers.
In comparison to traditional methods, the advantage of the BI-based puncture method proposed in this study lies in its consistent determination of the puncture reference points. This approach supersedes traditional anatomical landmarks (28) and has improved accuracy. Additionally, by adhering to the mathematical principles of the BI method, the selection of the puncture point becomes more flexible, allowing physicians to customize the procedure according to each patient’s anatomical features. Theoretically, this personalized approach should enhance puncture accuracy and elevate the level of individualized care.
The perpendicularity of the normal vectors of the two constructed planes, which indicates that the two planes intersect at a right angle, needs to be verified. It should be noted that the perpendicularity of the normal vectors does not directly indicate that the puncture trajectory is perpendicular to the skull (29). In theory, the line of intersection of two intersecting planes can be considered the puncture path. However, within the geometric space, the intersection line can uniquely determine the puncture trajectory only when the two planes are perpendicular to each other. In cases in which the planes are not perpendicular, the angle formed by their normal vectors diverges from 90°, thus introducing complexity into spatial transformation calculations, particularly those involving rotations and translations. Consequently, this increases the difficulty of making real-time adjustments to the puncture path during the procedure, which could lead to deviations from the intended direction.
Limitations
First, due to the relatively small sample size of this study, as well as inherent individual variations encountered during the puncture procedure, there may be some error associated with the calculated mean values. Studies with larger sample sizes need to be conducted to enhance the precision of the results. Second, our study, was based on the head CT scans of 135 adults, which limits the generalizability of our results to all age groups in the Chinese population, especially infants and children undergoing FV procedures. Extensive research is thus necessary to establish a comprehensive ventricle system database that accurately represents the pediatric population.
Conclusions
This study introduced a novel puncture method, referred to as the BI-based puncture method, which was developed using 3D model reconstruction technology, and is based on the principle of intersecting lines of two mutually perpendicular planes. The simulation results revealed that this novel method had superior accuracy and a higher optimal puncture rate than the traditional method. However, to comprehensively validate the effectiveness of this approach, further clinical trials need to be conducted. The application of the BI principle provides a new perspective for the analysis of brain puncture surgical techniques.
Acknowledgments
The authors would like to thank the other radiologists for their assistance with the data collection.
Footnote
Reporting Checklist: The authors have completed the STROBE reporting checklist. Available at https://qims.amegroups.com/article/view/10.21037/qims-24-1381/rc
Funding: This study was supported by funding from
Conflicts of Interest: All authors have completed the ICMJE uniform disclosure form (available at https://qims.amegroups.com/article/view/10.21037/qims-24-1381/coif). The authors have no conflicts of interest to declare.
Ethical Statement: The authors are accountable for all aspects of the work in ensuring that questions related to the accuracy or integrity of any part of the work are appropriately investigated and resolved. The study was conducted in accordance with the Declaration of Helsinki (as revised in 2013). The study was approved by the Ethics Committee of Fuzhou University Affiliated Provincial Hospital (No. K2020-06-019), and the requirement of individual consent for this retrospective analysis was waived.
Open Access Statement: This is an Open Access article distributed in accordance with the Creative Commons Attribution-NonCommercial-NoDerivs 4.0 International License (CC BY-NC-ND 4.0), which permits the non-commercial replication and distribution of the article with the strict proviso that no changes or edits are made and the original work is properly cited (including links to both the formal publication through the relevant DOI and the license). See: https://creativecommons.org/licenses/by-nc-nd/4.0/.
References
- Dey M, Jaffe J, Stadnik A, Awad IA. External ventricular drainage for intraventricular hemorrhage. Curr Neurol Neurosci Rep 2012;12:24-33. [Crossref] [PubMed]
- Saladino A, White JB, Wijdicks EF, Lanzino G. Malplacement of ventricular catheters by neurosurgeons: a single institution experience. Neurocrit Care 2009;10:248-52. [Crossref] [PubMed]
- Yuen J, Selbi W, Muquit S, Berei T. Complication rates of external ventricular drain insertion by surgeons of different experience. Ann R Coll Surg Engl 2018;100:221-5. [Crossref] [PubMed]
- Abdoh MG, Bekaert O, Hodel J, Diarra SM, Le Guerinel C, Nseir R, Bastuji-Garin S, Decq P. Accuracy of external ventricular drainage catheter placement. Acta Neurochir (Wien) 2012;154:153-9. [Crossref] [PubMed]
- De Backer A. Handbook of neurosurgery, 8th edition. Acta Chir Belg 2016;116:269.
- Hildebrandt G, Surbeck W, Stienen MN. Emil Theodor Kocher: the first Swiss neurosurgeon. Acta Neurochir (Wien) 2012;154:1105-15; discussion 1115. [Crossref] [PubMed]
- Muirhead WR, Basu S. Trajectories for frontal external ventricular drain placement: virtual cannulation of adults with acute hydrocephalus. Br J Neurosurg 2012;26:710-6. [Crossref] [PubMed]
- Toma AK, Camp S, Watkins LD, Grieve J, Kitchen ND. External ventricular drain insertion accuracy: is there a need for change in practice? Neurosurgery 2009;65:1197-200; discussion 1200-1. [Crossref] [PubMed]
- Foreman PM, Hendrix P, Griessenauer CJ, Schmalz PG, Harrigan MR. External ventricular drain placement in the intensive care unit versus operating room: evaluation of complications and accuracy. Clin Neurol Neurosurg 2015;128:94-100. [Crossref] [PubMed]
- Hsieh CT, Chen GJ, Ma HI, Chang CF, Cheng CM, Su YH, Ju DT, Hsia CC, Chen YH, Wu HY, Liu MY. The misplacement of external ventricular drain by freehand method in emergent neurosurgery. Acta Neurol Belg 2011;111:22-8.
- Kakarla UK, Kim LJ, Chang SW, Theodore N, Spetzler RF. Safety and accuracy of bedside external ventricular drain placement. Neurosurgery 2008;63:ONS162-6; discussion ONS166-7. [Crossref] [PubMed]
- Park YG, Woo HJ, Kim E, Park J. Accuracy and Safety of Bedside External Ventricular Drain Placement at Two Different Cranial Sites: Kocher's Point versus Forehead. J Korean Neurosurg Soc 2011;50:317-21. [Crossref] [PubMed]
- Olson DM, Zomorodi M, Britz GW, Zomorodi AR, Amato A, Graffagnino C. Continuous cerebral spinal fluid drainage associated with complications in patients admitted with subarachnoid hemorrhage. J Neurosurg 2013;119:974-80. [Crossref] [PubMed]
- Manfield JH, Yu KKH. Real-time ultrasound-guided external ventricular drain placement: technical note. Neurosurg Focus 2017;43:E5. [Crossref] [PubMed]
- Patil V, Gupta R, San José Estépar R, Lacson R, Cheung A, Wong JM, Popp AJ, Golby A, Ogilvy C, Vosburgh KG. Smart stylet: the development and use of a bedside external ventricular drain image-guidance system. Stereotact Funct Neurosurg 2015;93:50-8. [Crossref] [PubMed]
- Sarrafzadeh A, Smoll N, Schaller K. Guided (VENTRI-GUIDE) versus freehand ventriculostomy: study protocol for a randomized controlled trial. Trials 2014;15:478. [Crossref] [PubMed]
- Shtaya A, Roach J, Sadek AR, Gaastra B, Hempenstall J, Bulters D. Image guidance and improved accuracy of external ventricular drain tip position particularly in patients with small ventricles. J Neurosurg 2019;130:1268-73. [Crossref] [PubMed]
- Wilson TJ, Stetler WR Jr, Al-Holou WN, Sullivan SE. Comparison of the accuracy of ventricular catheter placement using freehand placement, ultrasonic guidance, and stereotactic neuronavigation. J Neurosurg 2013;119:66-70. [Crossref] [PubMed]
- AlAzri A, Mok K, Chankowsky J, Mullah M, Marcoux J. Placement accuracy of external ventricular drain when comparing freehand insertion to neuronavigation guidance in severe traumatic brain injury. Acta Neurochir (Wien) 2017;159:1399-411. [Crossref] [PubMed]
- Brenke C, Fürst J, Katsigiannis S, Carolus AE. High accuracy of external ventricular drainage placement using anatomical landmarks. Neurochirurgie 2020;66:435-41. [Crossref] [PubMed]
- Fisher B, Soon WC, Ong J, Chan T, Chowdhury Y, Hodson J, White A. Is Image Guidance Essential for External Ventricular Drain Insertion? World Neurosurg 2021;156:e329-37. [Crossref] [PubMed]
- Amoo M, Henry J, Javadpour M. Common Trajectories for Freehand Frontal Ventriculostomy: A Systematic Review. World Neurosurg 2021;146:292-7. [Crossref] [PubMed]
- Kirkman MA, Muirhead W, Sevdalis N. The relative efficacy of 3 different freehand frontal ventriculostomy trajectories: a prospective neuronavigation-assisted simulation study. J Neurosurg 2017;126:304-11. [Crossref] [PubMed]
- Raabe C, Fichtner J, Beck J, Gralla J, Raabe A. Revisiting the rules for freehand ventriculostomy: a virtual reality analysis. J Neurosurg 2018;128:1250-7. [Crossref] [PubMed]
- Lee KS, Zhang JJY, Bolem N, Leong ML, Goh CP, Hassan R, Salek AAM, Sein Lwin APT, Teo K, Chou N, Nga V, Yeo TT. Freehand Insertion of External Ventricular Drainage Catheter: Evaluation of Accuracy in a Single Center. Asian J Neurosurg 2020;15:45-50. [Crossref] [PubMed]
- Yi Z, He B, Deng Z, Liu Y, Huang S, Hong W. A virtual reality-based data analysis for optimizing freehand external ventricular drain insertion. Int J Comput Assist Radiol Surg 2021;16:269-76. [Crossref] [PubMed]
- Park B, Han S, Byoun HS, Han S, Choi SW, Lim J. The Assessment of Geometric Reliability of Conventional Trajectory of Ventriculostomy in a Three Dimensional Virtual Model and Proposal of a New Trajectory. Neurol Med Chir (Tokyo) 2020;60:264-70. [Crossref] [PubMed]
- Tavakoli S, Peitz G, Ares W, Hafeez S, Grandhi R. Complications of invasive intracranial pressure monitoring devices in neurocritical care. Neurosurg Focus 2017;43:E6. [Crossref] [PubMed]
- Stuart MJ, Mehigan B, Colbran RE, Withers TK, Ng W. Orthogonal external ventricular drain (EVD) trajectory from burr holes sited by junior neurosurgical staff is superior to freehand placement: An in-silico model. J Clin Neurosci 2021;94:65-9. [Crossref] [PubMed]


